Method

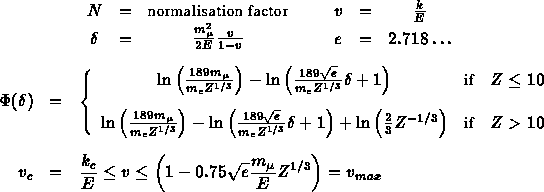
The differential cross-section for the emission of a photon of energy k by a muon of energy E is [,]:

$\{d\; \sigma dv\}=\; \{Nv\}(\; \{43\}-\{43\}v+v2)\; \Phi (\; \delta )$

where

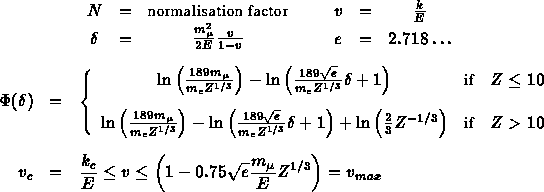
Therefore, the differential cross-section can be written as $\{d\; \sigma dv\}=\; f(v)\; g(v)$

with

$f(v)$	$=$	$[v\; ln(\; \{v$maxvc})]-1
$g(v)$	$=$	$\{1\Phi (0)\}(1-v\; +\{34\}v2)\Phi (\delta )$

We can sample the photon energy in the following way ($r$₁ , $r$₂ uniformly distributed random numbers in $]0,1[$ ):

1. sample v:

   $v=\; v$_c({v_maxv_c})^r₁

2. compute the rejection function $g(v)$ and:
   1. if $r$₂> g(v) go back to step 1
   2. if $r$₂≤g(v) , accept v and k = v E.

After the successful sampling of k, GBREMM generates the polar angles of the radiated photon with respect to an axis defined along the parent muon's momentum calling GBTETH. For more information on the sampling procedure see [PHYS341].

PHYS450